Introduction
The frequency response is a representation of the system's response to sinusoidal inputs at varying frequencies. The output of a linear system to a sinusoidal input is a sinusoid of the same frequency but with a different magnitude and phase. The frequency response is defined as the magnitude and phase differences between the input and output sinusoids.
The frequency response comprises of two parts:
1. Gain Response
2.Phase Response
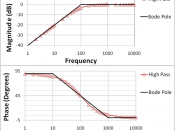
The frequency response of a system can be viewed two different ways: via the Bode plot or via the Nyquist diagram. Both methods display the same information; the difference lies in the way the information is presented. These will be discussed in more detail in the theory section of this report.
The circuit design is shown below:
Circuit: Functional Role of the Circuit
The circuit can be viewed as a potential divider network.
Shown in Fig 2
The reactance of the capacitor is given as
The circuit as a low pass filter.
A first order, low pass RC filter is simply an RC series circuit across the input, with the output taken across the capacitor. We assume that the output of the circuit is not connected, or connected only to high impedance, so that the current is the same in both R and C.
The voltage across the capacitor is IXC = I/wC. The voltage across the series combination is IZRC = I(R2 + (1/wC)2)1/2, so the gain is
From the phasor diagram for this filter, we see that the output lags the input in phase.
At the angular frequency w = wo = 1/RC, the capacitive reactance 1/wC equals the resistance R. We show this characteristic frequency on all graphs on this page. For instance, if R = 1 kW and C = 0.47 mF, then 1/RC...