Vectors Addition 9/24/2014
Chris Coutu
Justin Jones
Rajan Pandya
Quinn Howington
I. Introduction
The intent of this experiment is to add vectors effectively by using experimental, analytical, and
graphical methods; and to estimate random error from an instrument's measured sensitivity. The
method for vector addition includes finding the orthogonal components of each vector and adding
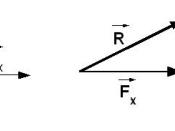
so that the components of the resultant vector R is related to the components of the individua l vectors A, B, etc., in the following way:
Rx = Ax + Bx Ry = Ay + By
The magnitude of the resultant vector and the angle θ between the vector R and the x-axis are as followed:
R = (Rx)2 + (Ry)2
TanÎ= [(Ry) / (Rx)]
The equilibrant vector is the vector E which is in opposite direction to vector R.
II. Materials and Methods
In the experiment, a force table with movable pulleys on its rim was used to determine the sum of
two given forces. Begin by the forces are being exerted on an object by two vectors at angles of
(450) and (1350), and, these forces are each equivalent to the weight of a (150) gram mass
(including 50 gram mass of weight hangers). The value of the resultant vector R and its direction
were calculated using the given formulas. On a graph paper, a scale graph was made of two vector
forces, one resultant vector force, and one equilibrium vector force. The force table was used to
measure the weight needed to exactly offset the resultant of your vectors. Thus, an equilibr ium
vector E with mass (210) gram at (2690) angle can be achieved. By repeating all these steps for
case 2, to add the vectors exerted by a (100) gram mass at (1630) and (200) gram at (2870); another
equilibrium vector E with mass (170) gram at (760) angle can be achieved. In analytical method,
to get the resultant vector R, addition of x-components of vector A and B, and addition of y-
components of vector A and B was done. For case 1, Rx and Ry are (0) and (212) respectively, and
for case 2 Rx and Ry are (-37.16) and (-162.02). The direction of the resultant vector will always be on opposite of the equilibrium vector.
III. Results and Discussion
In this experiment, the intent was to add vectors with graphical, experimental, and analyt ica l
methods. Adding vectors by component method proved to be more accurate than graphical method
because there were known values that were used. The other intent was to estimate random error
from instrument's measured sensitivity. Sensitivity in mass was (5) gram in each case, and
sensitivity in direction was +/- 0.50 in each case.
Questions-Answers
1. There is only one equilibrant vector E which balances other vectors, that is, there is only one
mass and angle which balances the resultant. It's because there are no errors in ideal world. In real world, because of number of errors, we can get more than one equilibrant vector.
2. Estimated random error in angle = +/- 0.50
Estimated random error in mass = +/- 5 gram
Pulleys and centering ring with three strings could cause this range in angles and masses that do not seem to affect the balance.
3. Instrumental error = 0.50
4. A "tug-of-war" can't be conducted with a pair of steel pipes welded to a wheeled safe to keep
the pipes' angle θ constant because it will keep moving the wheel in the direction of the resultant force. To stop that, it will need a equilibrant force to stop wheel from moving.
5.
100(Rexp - Rana)/Rana 100(Thetaexp - Thetaana)/Thetaana
Case 1 -0.9% -0.4%
Case 2 2.3% -1.4%
- I. Introduction
- II. Materials and Methods