Today, money has value when it is placed in interest-bearing accounts. When one wishes to meet a specific goal, the time attached to these accounts is a factor to consider. Annuities are like contracts in the sense that one agrees to them for a number of years. Choosing an annuity can be difficult, given that one should weigh the following factors: interest rates, compounding, present value, future values, opportunity cost, and the rule of '72.
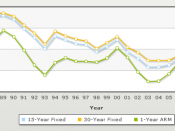
Interest Rates and CompoundingConsider the following example. I am looking for a loan in order to buy a house for $200,000. I prefer low interest rates, and I am not sure whether I should go with a fixed interest rate at 30 or 15 years. I got a quote for a 30-year fixed-rate loan at 8.5% and a 15-year fixed-rate loan at 5.9%. The payments would be as follows:_______200,000________30-year loan:Mortgage Payment = 360 Month Annuity Factor___200,000_____Mortgage Payment = (1.0825)360
English: Mechanism of Fixed Exchange Rate System
Cash
My Mortgage Docs to be Reviewed by an Expert= $1,537.83______200,000_________15-year loan:Mortgage Payment = 180 Month Annuity Factor = $1,676.93Future Value (of an Investment)The mortgage payments for the 30-year loan would be $139.13 lower than the payments for the 15-year loan. Upon realizing this, I wanted to determine whether it would be more advantageous to pay $139.13 more at a lower interest rate or to save $139.13 each month in an account. With an annual payment of $1,669.56 at a 10% interest rate for 15 years, how much would I earn? I needed to answer this question to compare my mortgage-loan options. I used a financial calculator to solve the problem. I entered 15 = N, 10 = I/Y, 0 = PV, $1,669.56 for the payment, and CPT FV = $53,046.06.
Annuities and the Rule of '72I now know that I could profit if I were to save the difference between the two mortgage payments. However, the profit is not enough for me to choose the 30-year fixed-rate loan. How long would it take me to double $53,046.06? Using the rule of '72, one takes 72/r, with r representing the rate. The results show how many years it would take to double the amount. If I got a low rate of 6%, I would take 72 divided by 6, showing that it would take 12 years to double.
Present Value (of a Future Payment Received)How much do I need to have to earn $251,771.40? This amount is the difference in interest in dollars that that I would be paying if I decided to take the 30-year loan. If I got a loan for 15 years at 10%, how much would I need to save? I would need $60,272.07 in the bank so that I could earn $251,771.40.
____$251,771.40___PV = (1+.010)15 = 60,272.07Opportunity CostWhat would the $200,000, 30-year fixed-rate loan cost in terms of interest? The answer is $353,618.80. Wow! The 15-year loan would cost $101,847.40 in interest. The 30-year loan payment would be lower, but the total interest I would pay would be much greater. The 15-year loan would save me $251,771.40 in interest, though the monthly payment would be $139.13 more.
Getting the most for one's dollars is important. Using all of the TVM formulas has helped me to decide which mortgage loan to choose. The 15-year loan is my choice. Paying a higher payment at a lower interest rate will save me $251,771.40-a significant amount of money. I will also own my home in 15 years rather than 30 years.
ReferencesBeasley, R. A., Myers, S. C., & Marcus, A. K. (2003). Fundamentals of corporate finance (4th ed.). Publication City: McGraw-Hill.
Ekman, P. D. (2005). Disk lectures. Retrieved Month Day, Year, fromhttp://www.disklectures.com/freebies.phpTechSmith. (2006). SnagIt (Version 8.2.0) [Computer software]. Retrieved fromhttp://www.techsmith.com/download/snagittrial.asp
English: Mortgage rates historical trends
Mortgage Loan Fraud Assessment based upon Suspicio...
buried cash