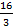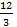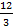Mathematical Investigation:
VON KOCH'S SNOWFLAKE CURVE
Introduction:
History of Von Koch's Snowflake Curve
The Koch snowflake is a mathematical curve, which is believed to be one of the earliest fractal curves with description. In 1904, a Swedish mathematician, Helge von Koch introduced the construction of the Koch curve on his paper called, "On a continuous curve without tangents, constructible from elementary geometry".
In this mathematical task, I am going to investigate how the area and perimeter of a shape/curve changes and find out whether they increase by the same number every time,as the following process is repeated:
Start with an equilateral triangle.
Divide each side of the triangle into three equal segments.
On the middle part of each side, draw an equilateral triangle by connecting lines.
Now remove the line segment that makes the base of the smaller triangle that was formed in step 3.
The above process (steps i~iv) can be repeated indefinitely.
The shape that emerges is called "Von Koch's Snowflake" for obvious reasons. An equilateral triangle, which is the shape used to start with to draw the Koch Snowflake curve, turns its shape similar to a star or a snowflake as each side of the previous curve is pushed out.
Process:
In this investigation, the process of drawing the Koch curve has to repeat in order to generalize rules for both perimeter and area.
Perimeter: Under the assumption that the equilateral triangle (so-called C0) at the very start has a perimeter of 3 units, find the perimeter for the next curves (C1, C2, C3, and so on), and eventually, find the perimeter of Cn.
During the second iteration, when extra equilateral triangles are added on the middle part of each side of the new curve, C1, the perimeter increases to